Die Laplace-Matrix ist in der Graphentheorie eine Matrix, welche die Beziehungen der Knoten und Kanten eines Graphen beschreibt. Sie wird unter anderem zur Berechnung der Anzahl der Spannbäume und zur Abschätzung der Expansivität regulärer Graphen benutzt. Sie ist eine diskrete Version des Laplace-Operators.
Laplace-Matrizen und insbesondere ihre zu kleinen Eigenwerten gehörenden Eigenvektoren werden beim Spectral Clustering, einem Verfahren der Clusteranalyse, verwendet.
Definition
Die Laplace-Matrix  eines Graphen mit der Knotenmenge
eines Graphen mit der Knotenmenge  und der Kantenmenge
und der Kantenmenge  ist eine
ist eine  Matrix. Sie ist definiert als
Matrix. Sie ist definiert als  , wobei
, wobei  die Gradmatrix und
die Gradmatrix und  die Adjazenzmatrix des Graphen bezeichnet. Der den Knoten
die Adjazenzmatrix des Graphen bezeichnet. Der den Knoten  und
und  entsprechende Eintrag ist also
entsprechende Eintrag ist also
 .
.
Die Grad-Matrix ist eine Diagonalmatrix und hat im Eintrag  die Zahl der Kanten, welche im Knoten
die Zahl der Kanten, welche im Knoten  enden.
enden.
Insbesondere ist die Laplace-Matrix eines  -regulären Graphen
-regulären Graphen

mit der Einheitsmatrix  .
.
Beispiel
| Nummerierung der Ecken | Gradmatrix | Adjazenzmatrix | Laplace-Matrix |
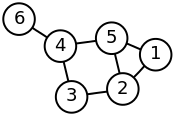 |  |  |  |
Zusammenhang mit Inzidenzmatrix
Die Laplace-Matrix kann auch durch die Inzidenzmatrix berechnet werden. Sei  eine
eine  Inzidenzmatrix, dann ist die Laplace-Matrix gegeben durch
Inzidenzmatrix, dann ist die Laplace-Matrix gegeben durch
 .
.
Eigenschaften
Wir bezeichnen mit  die Eigenwerte der Laplace-Matrix, siehe Spektrum (Graphentheorie).
die Eigenwerte der Laplace-Matrix, siehe Spektrum (Graphentheorie).
 ist symmetrisch.
ist symmetrisch. ist positiv-semidefinit, insbesondere also
ist positiv-semidefinit, insbesondere also  für alle
für alle  .
. ist eine M-Matrix.
ist eine M-Matrix.- Die Spalten- und Zeilensummen sind Null. Insbesondere ist
 mit Eigenvektor
mit Eigenvektor  .
. - Die Vielfachheit des Eigenwertes
 ist die Anzahl der Zusammenhangskomponenten des Graphen.
ist die Anzahl der Zusammenhangskomponenten des Graphen.




































